Application of ultra-high gravity wall in high fill slope engineering on karst developed foundation
-
摘要:
重庆武隆机场南端西侧岩溶发育、地形陡峻,采用了高路堤与超高衡重式挡墙相结合的高填方边坡方案。挡墙基础发育3处岩溶,面积占挡墙的45%以上,全填充,最大深度超过30 m,属于典型的特殊复杂地基。为了解决岩溶地基不均匀性强、承载力低、边坡及挡墙稳定性问题突出等难题,采用开挖一定深度的岩溶充填物并回填混凝土方案。通过理论计算详细分析了不同换填深度下高边坡及高挡墙的破坏模式、稳定性、应力及变形规律,确定了合理的岩溶换填深度。研究结果表明,采用一定深度的岩溶换填方案可有效改善岩溶地基的不均匀性,降低挡墙应力集中效应,减小挡墙及高填方变形,大幅提高挡墙及边坡稳定性。现场监测表明,高挡墙及高边坡工后水平和竖向位移均小于4 mm,变形曲线收敛,边坡及挡墙稳定性良好。研究成果对于复杂山区高填方工程规划设计及施工具有重要的参考意义。
Abstract:This paper presents a high fill slope solution for the steep and karst-developed terrain at the southern end west side of Wulong Airport in Chongqing, where a combination of high embankment and ultra-high counterweight retaining wall was adopted. The foundation of the gravity wall consists of three karst areas, which accounts for more than 45% of the total area. These karst areas are fully filled and have a maximum depth exceeding 30 m, making them typical examples of special and complex foundations. To address challenges such as strong non-uniformity, low bearing capacity, and instability of slopes and retaining walls in karstic foundations, a solution involving excavation to a certain depth in the karst and backfilling with concrete was adopted. Through theoretical calculations, this paper comprehensively analyzes the failure modes, stability, stress and deformation of the high slope and retaining wall with varying concrete replacement depths, ultimately determining a suitable replacement depth. The research results show that the adoption of a certain depth of replacement can effectively improve the non-uniformity of karst foundations, reduce stress concentration on the retaining wall, decrease deformation of the retaining wall and high embankment, and significantly enhance the stability of the retaining wall and slope. Field monitoring indicates that the horizontal and vertical displacements of the high retaining wall and slope after construction are both less than 4 mm, and the deformation curve converges, demonstrating their good stability. The research findings have important reference significance for the planning, design, and construction of high-fill slope projects in complex mountainous areas.
-
Keywords:
- ultra-high gravity wall /
- karst /
- failure mode /
- high fill slope /
- stability /
- numerical simulation
-
0. 引言
降雨是滑坡失稳的主要诱发因素,土体裂隙为雨水提供了入渗通道,沿此通道形成的优势流主导着降雨入渗过程,导致滑坡稳定性逐步恶化,促使变形持续发展[1 − 4]。因此,在评价滑坡稳定性时,能够正确认识优势流的入渗过程非常重要。
近年来关于优势流定量研究成为了热点问题。优势流对粘土质斜坡的孔隙水压力起着主要的控制作用[5]。在降雨触发滑坡的过程中,孔隙水压力、含水率等参数指标的变化受优势流入渗的影响[6]。在研究降雨诱发震后滑坡再复活的水力学特性时发现优势流主导着降雨入渗过程,并增加垂向渗透性能和孔隙水压力,加快地下水对降雨的响应,降低土壤有效应力,进而诱发滑坡复活[7]。通过模拟地表裂缝对滑坡水文动态变化特征的影响,发现裂缝的体积和连通性控制着滑坡中孔隙水压力的分布[8]。边坡变形破坏期间,优势流为地表水渗透和地下水运移提供了通道,进一步加速了边坡变形[9]。
在膨胀土滑坡的研究中,主要关注膨胀土的胀缩性与裂隙性造成的膨胀土强度的不均匀性和应力差异导致滑坡失稳[10 − 11],往往忽视了膨胀土的低渗透性,有关优势流入渗导致膨胀土滑坡的失稳机理研究较少。目前大多数的研究主要集中于笼统的均质降雨入渗进行研究,较少考虑裂隙对降雨入渗的影响,实际上,膨胀土斜坡上的细宏观变形(干缩裂隙、地表裂缝)对降雨入渗有重要影响,是诱发膨胀土滑坡发生失稳不可忽视的因素。
1. 研究方法
1.1 研究对象
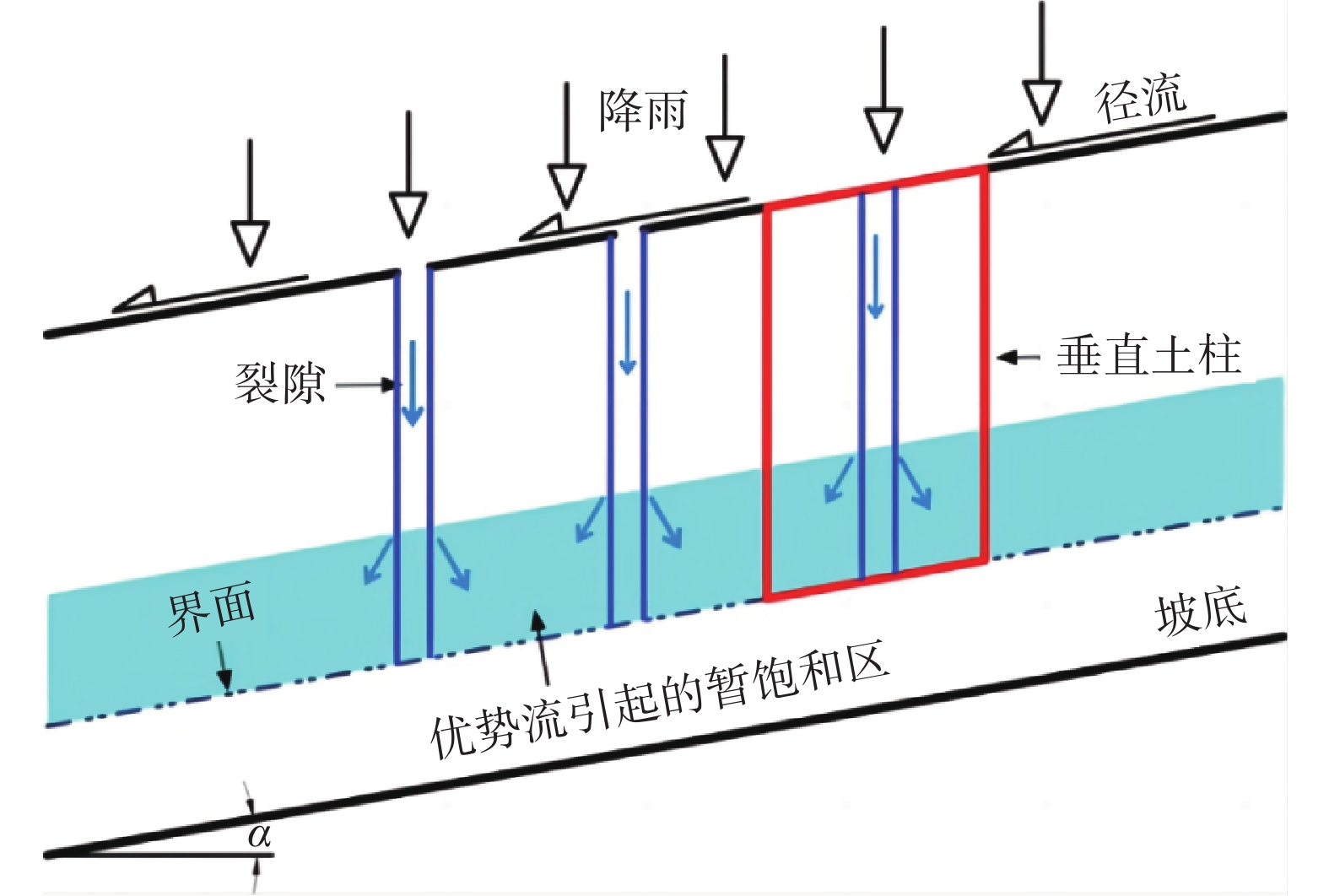
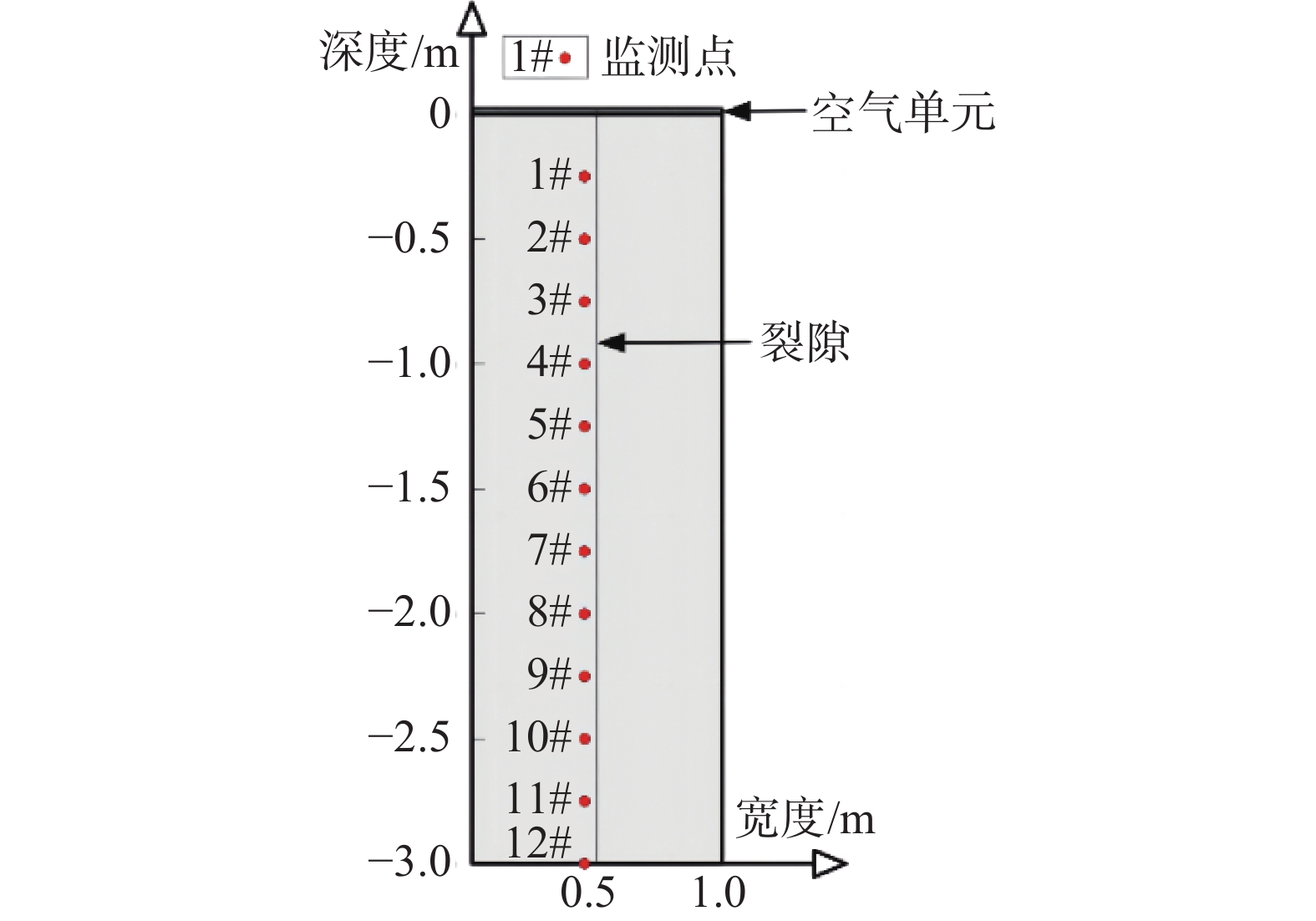
双重渗透介质模型可以较好地描述裂隙优势流、基质孔隙流以及裂隙-基质的流量交换[12 − 13]。Indrawan等人[14]通过实验室方法和数值模拟方法对两层土柱进行了一维入渗的全面研究,朱伟等[15]用自行研发的土柱装置结合数值模拟的方法深入探究了土壤的降雨入渗特征。本文将含裂隙坡体降雨入渗简化为含单一垂直裂隙的斜坡土柱模型进行研究,如图1中红色框所示。利用Richards方程基本理论,基于离散孔隙-裂隙介质模型,结合两种典型降雨工况,以不同裂隙深度为主要变量,探讨裂隙在发育演化过程中的优势流入渗特征。
1.2 计算理论
Richards方程是基于Darcy定律和质量守恒定律,是描述降雨入渗问题的严格数学物理模型,可以很好的模拟降雨入渗中水分在一维土体中运动的三个过程:即入渗、水分重分布和排水过程[16]。因此,裂隙与基质的非饱和渗流均采用Richards方程进行描述:
$$ ({C}_{\text{m}}+{S}_{{\mathrm{r}}}S)\frac{\partial h}{\partial t}-\nabla \cdot [{k}_{{\mathrm{m}}}(\nabla h+1)]=0 $$ (1) 式中:Cm——容水度;
Sr——饱和度;
S——贮存系数;
h——压力水头;
km——土体的渗透系数,
$ {k}_{{\mathrm{m}}}={{k}}_{\mathrm{s}}{{k}}_{\mathrm{r}}\left({{S}}_{\mathrm{r}}\right) $ ;ks——土体饱和渗透系数;
kr——基质相对渗透系数。
基质的相对渗透系数kr以及其他特征参数采用Van Genuchten(V-G)模型描述:
$$ {S}_{r}=\left\{\begin{split} &{\left(1+{\left|\alpha {H}_{p}\right|}^{n}\right)}^{-m}, &{H}_{p} < 0\\ &1, &{H}_{p}\geqslant 0\end{split}\right. $$ (2) $$ {C}_{m}=\left\{\begin{split} &{\left(\frac{am}{1-m}\left({\theta }_{s}-{\theta }_{r}\right){S}_{e}^{\tfrac{1}{m}}\left(1-{S}_{e}^{\tfrac{1}{m}}\right)\right)}^{m},& {H}_{p} < 0\\ &0, &{H}_{p}\geqslant 0\end{split}\right. $$ (3) $$ {k}_{r}=\left\{\begin{split} &{{S}_{e}^{\tfrac{1}{2}}\left[1-{\left(1-{S}_{e}^{\tfrac{1}{m}}\right)}^{m}\right]}^{-2}, &{H}_{p} < 0\\ &1, &{H}_{p}\ge 0\end{split}\right. $$ (4) 式中:α、n和m——模型拟合参数;
Hp——压力水头。
采用Brooks-Corey(BC)模型[17]描述裂隙的非饱和特性,其表达式为。
$$ {S}_{r}=\left\{\begin{split} &{\left|\alpha {H}_{p}\right|}^{-n}, &{H}_{p} < 1/\alpha \\ &1, &{H}_{p}\geqslant -1/\alpha \end{split}\right. $$ (5) $$ {C}_{m}=\left\{\begin{split} &\frac{-n}{{H}_{p}}\left({\theta }_{s}-{\theta }_{r}\right){\left|\alpha {H}_{p}\right|}^{-n},& {H}_{p} < 1/\alpha \\ &0, &{H}_{p}\geqslant -1/\alpha \end{split}\right. $$ (6) $$ {k}_{r}=\left\{\begin{split}&{S}_{e}^{2/n+5/2}, &{H}_{p} < -1/\alpha \\ &1, &{H}_{p}\geqslant -1/\alpha \end{split}\right. $$ (7) 假设裂隙由具有一定曲折度的粗糙平板组成,则雨水在裂隙中的渗流满足立方定律[18],裂隙的渗透系数
$ {k}_{f} $ 为:$$ {{{k}}_f} = C \cdot \frac{{gd_f^2}}{{12\mu }} $$ (8) 式中:C——裂隙曲折度;
g——重力加速度;
$ \mu $ ——流体的动力粘滞系数;基于K-C模型,通过对比曲折裂隙与平直裂隙之间的渗透性差异,得到裂隙曲折度C[19]:
$$ C = \frac{1}{{{\tau ^2}{T_{{\text{s}}}}^2}} $$ (9) 式中:
$ \tau $ ——孔隙曲折系数,$ \tau = \sqrt {1 - {\text{ln}}({n^2})} $ [20];n——土体孔隙率;
Ts——面曲折系数,本文考虑裂隙面具有一定起伏,取1.04[21]。
1.3 计算模型及参数
COMSOL Multiphysics基于有限元方法,通过求解部分单场或多场的偏微分方程,从而来模拟真实物理现象,在岩土力学领域中,COMSOL MultiphySics有限元软件可以通过高度准确的渗流稳定分析计算,已在双重介质渗流领域获得广泛应用[22 − 25]。
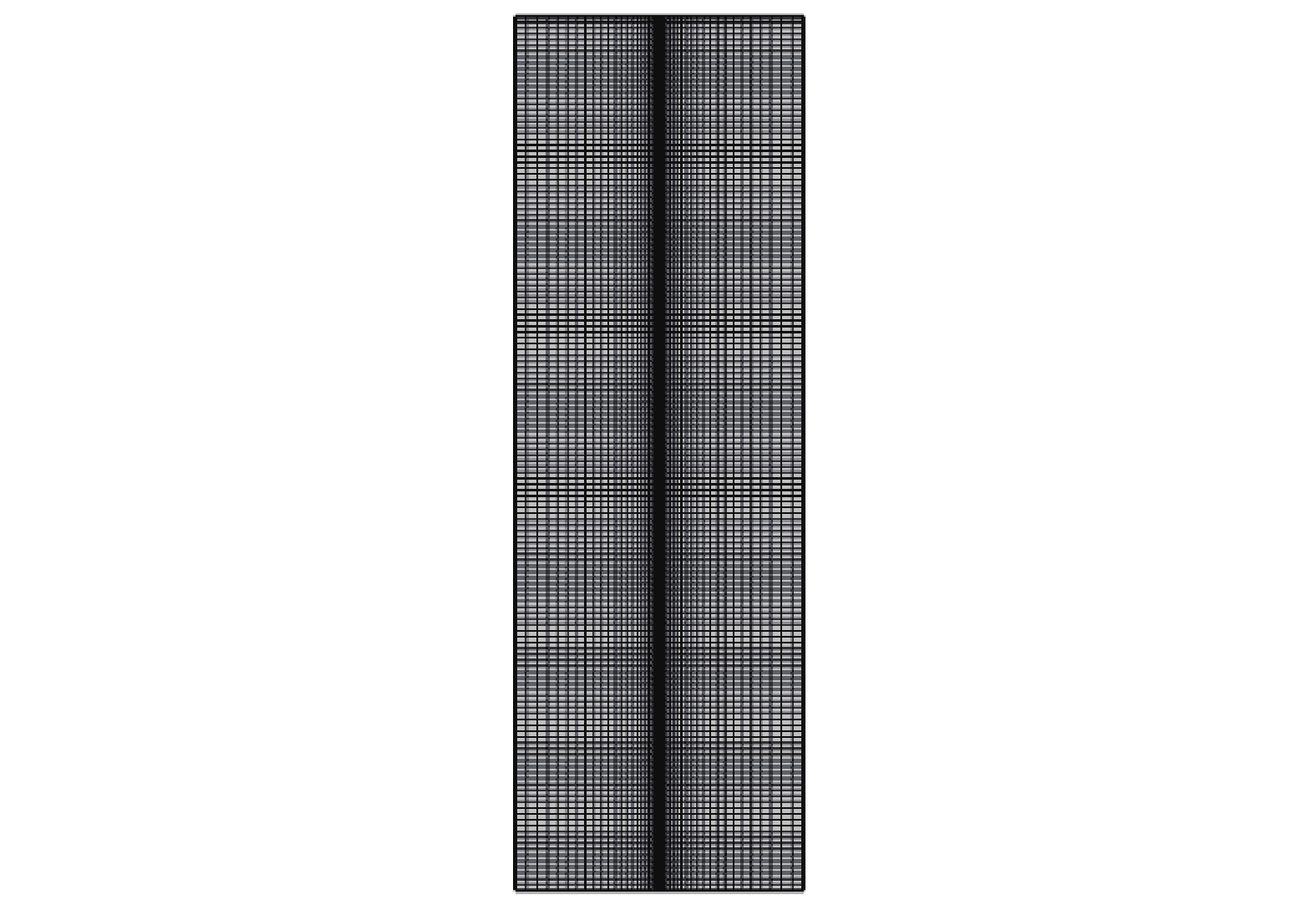
模型参照十堰市郧县某滑坡,建立高3 m,宽1 m的二维土柱模型,在土柱中部(即x=0.5 m处)设置一条垂直裂隙,如图2。采用有限单元分别离散裂隙和土体基质,模型中所有侧边界为不透水边界。上边界为降雨入渗边界,降雨入渗边界采用“空气单元”概念[26 − 27],通过监测表面压力以切换流量边界与边界。下边界为固定水头边界。模型共划分7800个节点和7599个单元,其中有裂隙单元149个和基质单元7400个,单元边长0.02 m。在裂隙附近对网格进行加密,与裂隙相邻的基质单元尺寸为0.002 m,远离裂隙的单元尺寸以等差数列逐渐增大至0.02 m,如图3。
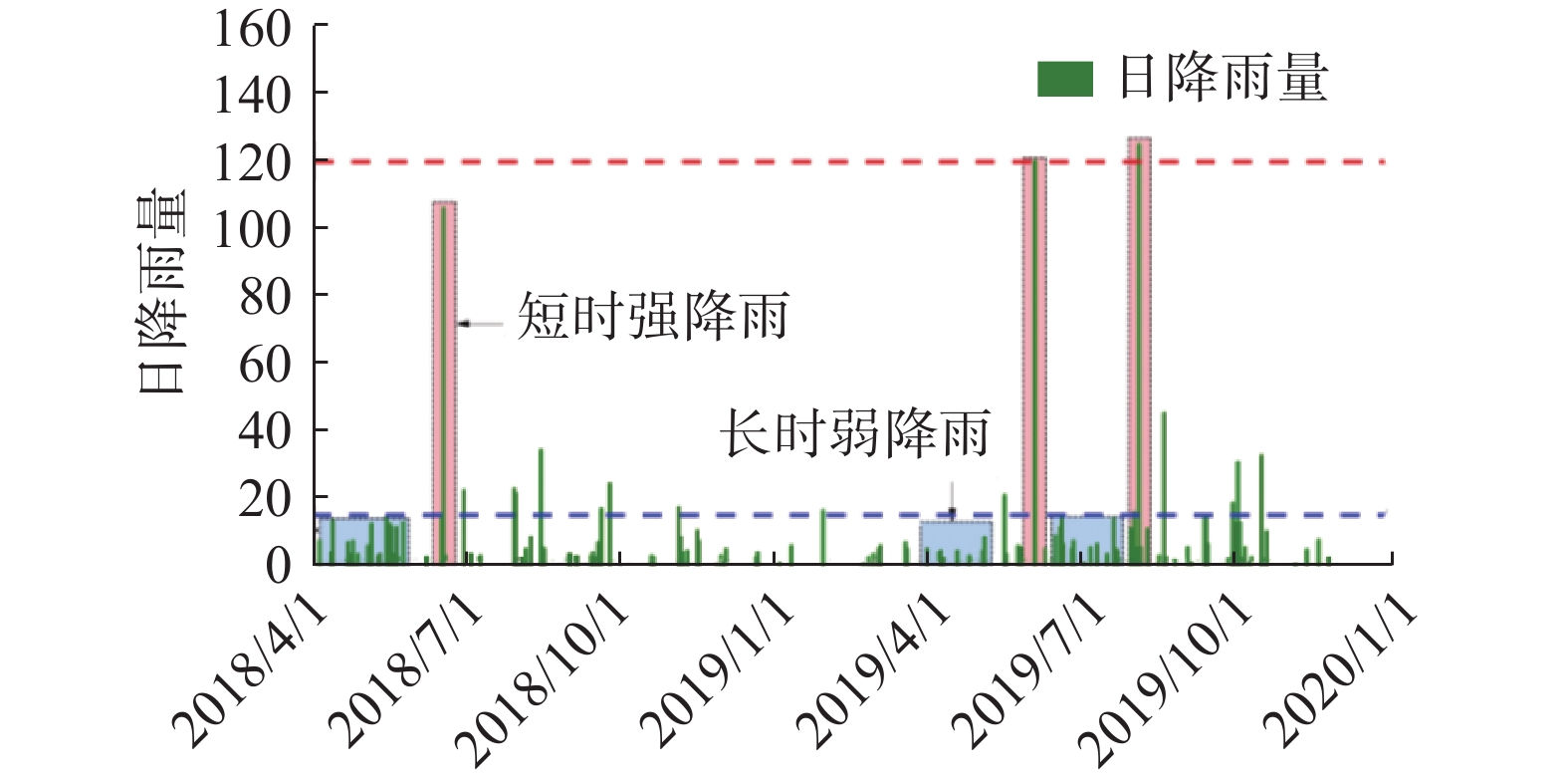
Lee 等[28]根据降雨特性将降雨分为短时强降雨和长时弱降雨两种情况,结合研究区的实际降雨情况(图4),将降雨工况设置为短时强降雨、长时弱降雨两种,考虑不同裂隙深度、不同裂隙宽度的优势流入渗特征,
$ {k}_{f} $ 根据式(8)得到。具体工况见表1。表 1 模拟工况Table 1. Simulated working conditions工况 编号 裂隙深度(m) 裂隙宽度(mm) $ {k}_{f} $(m/s) 降雨 短时
强降雨1 无裂隙 - - 120 mm/d,
持续48 h2 0.5 5 0.032 3 1 10 0.131 4 2 20 0.527 长时
弱降雨5 无裂隙 - - 12 mm/d,
持续480 h6 0.5 5 0.032 7 1 10 0.131 8 2 20 0.527 饱和渗透系数为现场试验结果,Van Genuchten(V-G)模型参数[29]、Brooks-Corey(B-C)模型参数[24]为经验取值,具体参数取值见表2。
表 2 模拟参数Table 2. Simulation parameters符号 名称 数值 来源 ks 饱和渗透系数 5.787×10−8 m/s 勘查资料 $ \alpha $ V-G模型本构参数 0.272 经验取值 $ n $ V-G模型本构参数 2.3 经验取值 θs 饱和含水率 32.6% 室内试验 θr 残余含水率 6.4% V-G模型拟合 $ \alpha $ B-C模型本构参数 6.8 经验取值 $ n $ B-C模型本构参数 1 经验取值 2. 数值计算结果
2.1 入渗过程
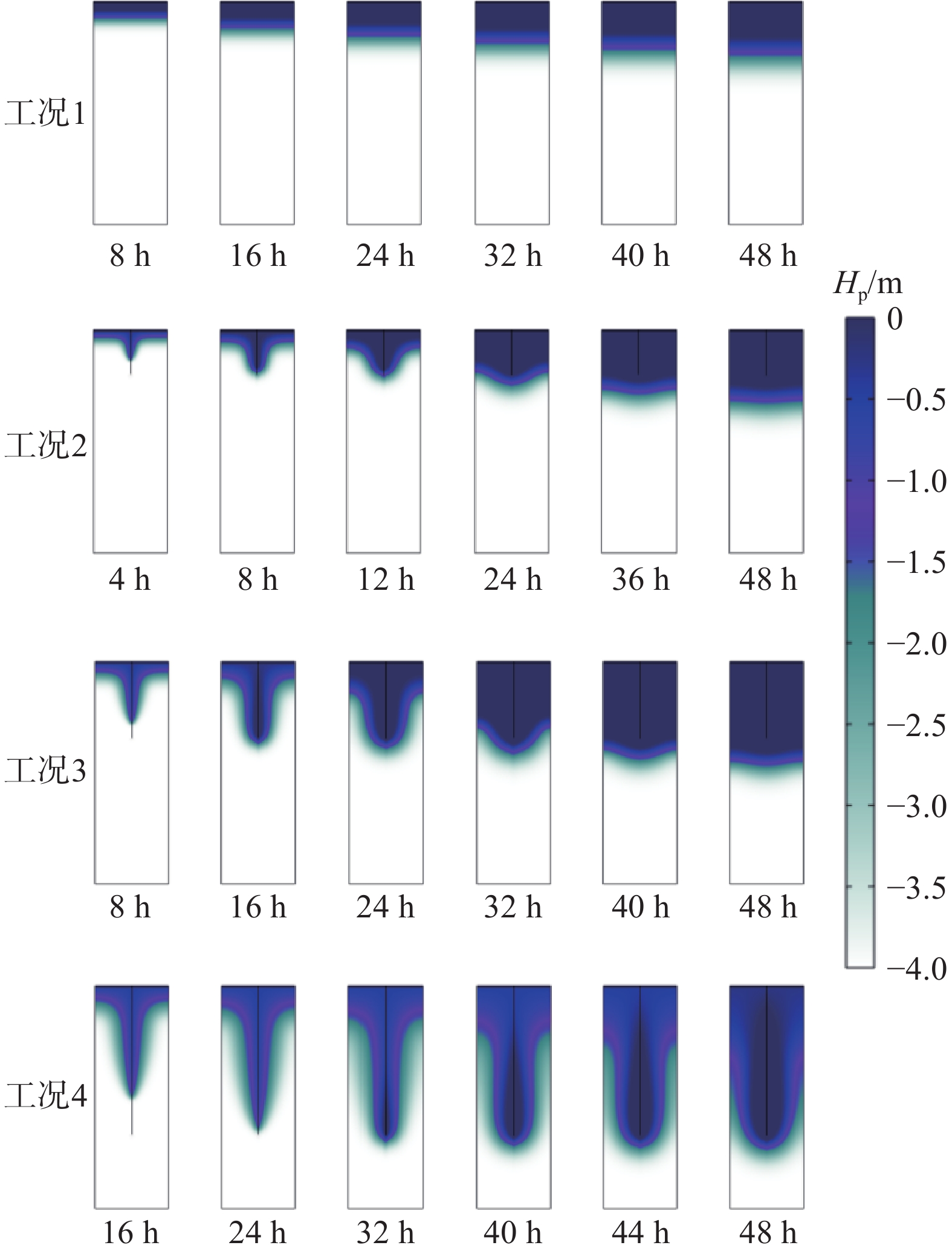
图5、6为不同工况下压力水头分布随降雨时间的变化过程。无裂隙时(工况1、工况5),降雨开始后,土柱表层迅速润湿,降雨均匀向下垂直入渗,浸润锋呈“直线型”,短时强降雨难以入渗到较深的位置,大部分雨水形成坡面径流流失,长时弱降雨入渗深度显著增加,最大影响深度达到2.5 m。
裂隙存在时,在短期强降雨下(工况2-4),降雨初期沿裂隙入渗,产生优势流。部分降雨从土柱表面均匀向下垂直入渗,部分沿裂隙入渗,入渗至裂隙末端,由于基质渗透系数较差,降雨在裂隙末端聚集形成局部暂饱和区,并向两侧水平入渗,入渗范围和最大影响深度随着裂隙发育而增加。浸润锋随降雨持续表现为“漏斗型”发展至“水滴型”最后发展至“直线型”,这是由于裂隙深度较短,土柱表面的饱和区与裂隙末端的饱和区更快连通,随着裂隙的深度增大,土柱表面的饱和区与裂隙末端的饱和区连通缓慢。
长时弱降雨下(工况6-8),在降雨初期(240 h内),四种工况浸润锋均呈“直线型”发展,优势流入渗现象不明显,表明雨水沿裂隙入渗速度与在基质孔隙入渗速度基本一致。随着降雨持续,雨水入渗至裂隙末端,在垂直入渗与水平入渗共同影响下,裂隙深度范围内土体迅速饱和。
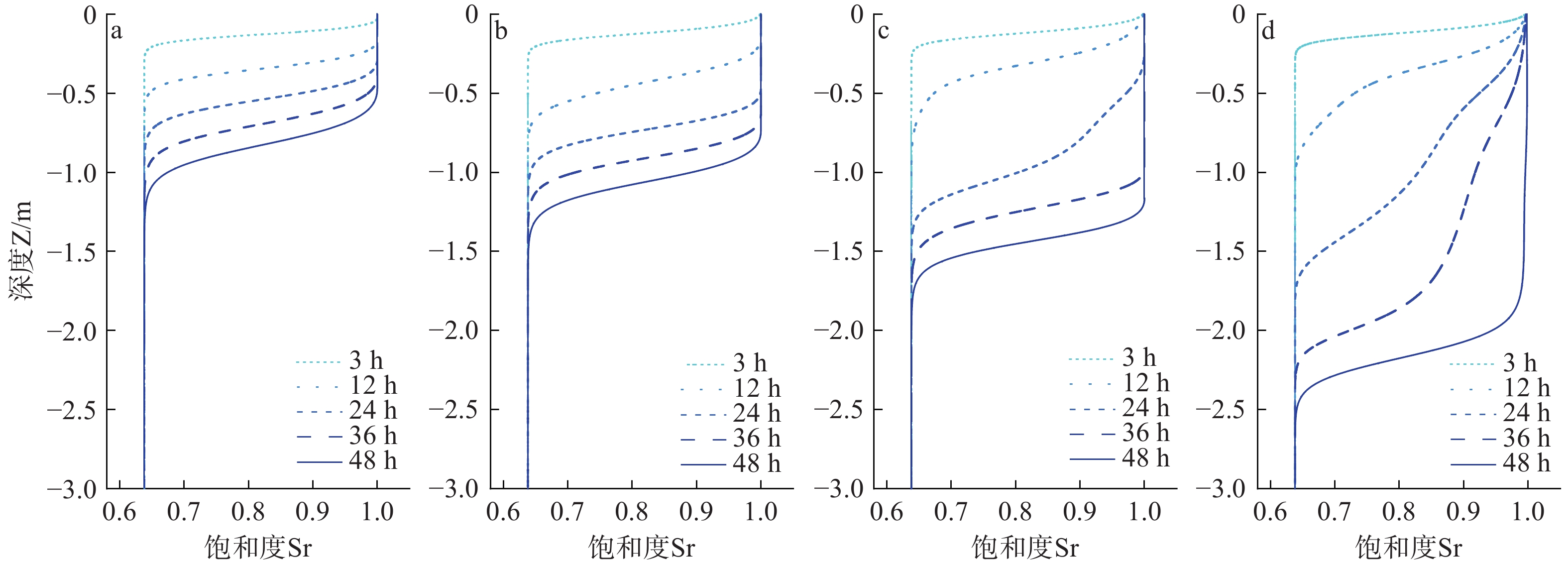
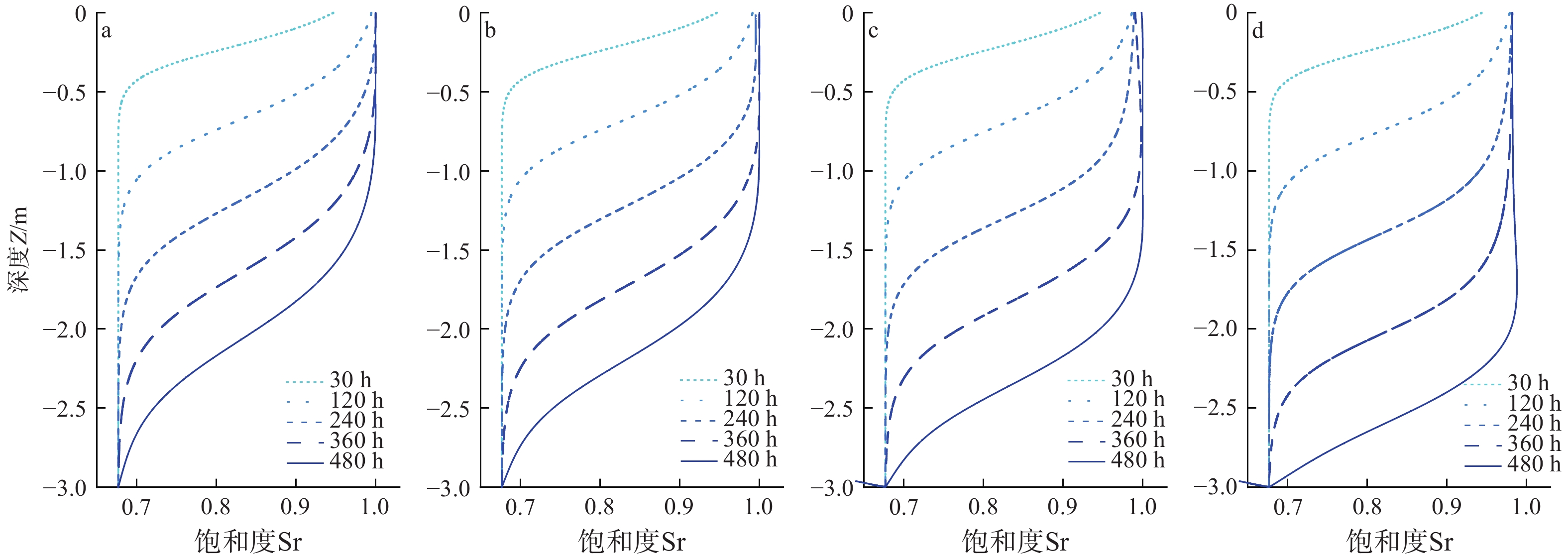
2.2 饱和度剖面
图7、8为不同工况下饱和度剖面随降雨时间的变化过程。在短时强降雨作用下,相同降雨时间内,降雨入渗深度随裂隙的出现与演化逐渐增加,无裂隙发育时,饱和度剖面随时间均匀增加。裂隙发育初期虽然饱和度总体呈均匀增加,但相较于无裂隙的情况,入渗深度显著增加。随着裂隙的进一步发育,饱和度开始不均匀增加,这是降雨在裂隙末端聚集并向两侧水平入渗导致的。
长时弱降雨作用下,几种工况的饱和度剖面随时间都均匀变化,在降雨480 h后饱和度剖面基本一致,入渗深度并没有随裂隙的出现与拓展明显增加,降雨入渗总体沿土柱表面均匀入渗。
2.3 入渗率
早期Green和Ampt作了以下假设:①湿润锋未到达的部分吸力水头为固定值不变,②湿润锋已经过的部分含水量和渗透系数也维持不变。则某一时刻时刻单位横截面积的总入渗位移q可以计算如下[30]:
$$ {{q}} = ({\theta _i} - {\theta _0})\textit{z} $$ (10) 式中:θi——湿润锋经过部分含水量;
θ0——湿润锋未到达部分含水量;
z——湿润锋经过的长度。
累积入渗量是降雨入渗到地下的总量:
$$ Q = A(\theta_i - {\theta _0})\textit{z} $$ (11) 若湿润锋后面土体含水率不为常量,它是随深度增加而不断发生变化的变量,则需对含水量的增加量在深度上进行积分。瞬时入渗率等于进水边界的入渗率i,可用达西定律表示为:
$$ i = \frac{{dQ}}{{Adt}} = \frac{{({\theta _i} - {\theta _0})d\textit{z}}}{{dt}} $$ (12) 图9、10为不同工况下入渗率随降雨时间的变化过程,短时强降雨作用下,几种工况监测点响应个数随裂隙出现与演化增多,并且入渗率达到稳定后,其数值于土体饱和渗透系数基本一致,表明入渗率达到稳定后,土体处于饱和状态。随着裂隙的出现与演化,各监测点响应时间与达到稳定的时间有所不同。工况1-工况4各监测点响应时间随深度增加而增加,表现为自上向下的顺序响应。
当无裂隙发育或裂隙发育初期(工况1-工况2),各监测点反应的入渗率虽然依次达到稳定,但是进一步对比这两种工况可以发现1#、2#监测点入渗率达到稳定的时间工况2远短于工况1,这是由于在裂隙发育初期,雨水快速入渗至裂隙末端形成暂饱和区,土体表层在基质诱导的垂直入渗与裂隙诱导水平入渗共同影响下迅速饱和,表现为1#、2#监测点在响应后迅速达到稳定。随着裂隙进一步发育(工况3-工况4),位于裂隙末端附近的监测点优先达到饱和,例如,工况3的3#监测点优先于1#、2#监测点达到饱和;工况4的7#、8#监测点优先于土柱表层其他监测点达到饱和,这表明随着裂隙的发育,降雨优势入渗逐渐明显,入渗过程由孔隙控制的垂直入渗转变为由裂隙控制的水平入渗。
长时弱降雨作用下,不同工况监测点响应个数和响应顺序基本一致(图10.a-d),总体表现为沿土柱表面均匀入渗。随裂隙出现,当降雨入渗至裂隙底部时,受基质垂直入渗和裂隙水平入渗的控制,裂隙末端附近入渗率迅速增大,裂隙影响范围内土体快速达到饱和状态。例如,工况6的2#监测点先于1#监测点达到饱和;工况7的4#、5#监测点优先于其他监测点达到饱和。
2.4 表面压力及入渗量
土壤总入渗量为基质入渗量与裂隙入渗量之和,假设土柱模型纵向厚度为1 m,对研究区域边界进行线积分,得到不同时刻下基质表面和裂隙表面的入渗量。短时强降雨工况下,如图11、12所示,工况1-工况4(图11.a-11.d)在降雨初期基质与裂隙的入渗能力均较强,降雨可以全部入渗,总入渗量均迅速增加,对应的表面压力急剧上升;随着裂隙的出现与演化,基质与裂隙的表面压力及入渗量随降雨时间的变化不同。当坡体表面无裂隙发育时,随着降雨持续,坡体表层逐渐饱和,此时降雨大部分转化为坡面径流,入渗边界主要为压力边界,入渗量受坡体本身透水能力控制,降雨开始后入渗量急剧增长至0.1 m3/d,孔隙水压力增大至0 kPa,并在后续时间内始终大于0,入渗量逐渐降低至稳定。
随着裂隙的出现与发育,在降雨持续入渗的过程中,入渗量的变化可以分为两个阶段:
①总入渗量处于上升阶段,该阶段基质入渗量逐渐减少,裂隙入渗量逐渐增加并超过基质入渗量,未产生坡面径流。随着降雨持续,裂隙表面压力出现“阶跃”,浸润锋从“漏斗型”转化为“水滴型”。随着裂隙发育演化,降雨稳定入渗的时间增加。②随着裂隙逐渐达到饱和,总入渗量处于下降阶段,基质和裂隙表面压力处于上升阶段。面径流产生,但此时裂隙影响范围内的土体未完全饱和,大部分降雨仍沿着裂隙入渗,裂隙表面压力仍小于0。随着裂隙影响范围内的土体饱和,裂隙表面压力大于0,总入渗量逐渐降低至稳定。
在长时弱降雨工况下,无裂隙发育时降雨入渗边界转换为压力边界的时间明显延长,降雨开始后入渗量显著增长,但对应的坡体表面孔隙水压力逐渐增大至0 kPa,随着降雨持续,总入渗量逐渐稳定。随着裂隙的出现与发育(工况6-工况8,图12.b-12.d),稳定入渗阶段时间延长,在裂隙H=1 m时,降雨持续时间内入渗量基本处于稳定入渗阶段,表面压力在持续时间内小于0,未产生坡面径流。
3. 优势流入渗规律
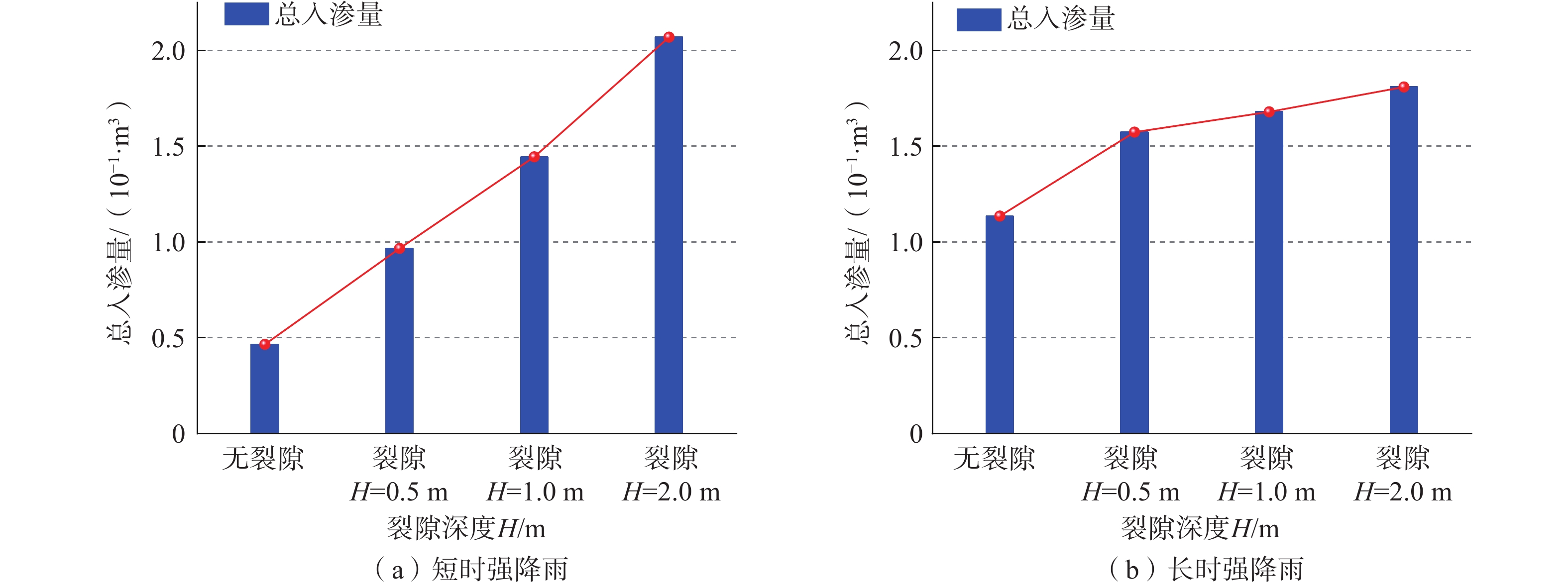
图13、14为2种典型降雨工况下的降雨入渗深度和总入渗量情况。短时强降雨作用下,无裂隙时入渗深度为0.45 m,总入渗量为0.46×10−1 m3。裂隙H=0.5 m、1.0 m、2.0 m时,入渗深度分别为0.72 m、1.16 m、2.11 m,总入渗量分别为0.96×10−1 m3、1.44×10−1m3、2.07×10−1 m3。相同降雨时间条件下,入渗深度和入渗量与裂隙深度呈正比,降雨入渗深度与裂隙深度基本一致。长时弱降雨作用下,无裂隙时入渗深度为1.31 m,总入渗量仅为1.13×10−1 m3。裂隙H=0.5 m、1.0m、2.0 m时,降雨480 h内,入渗深度分别为1.51 m、1.78 m、2.00 m,总入渗量分别为1.57×10−1 m3、1.68×10−1 m3、1.81×10−1 m3。随着裂隙出现与深度增加,其入渗量和入渗深度增幅较小,优势流作用不显著。
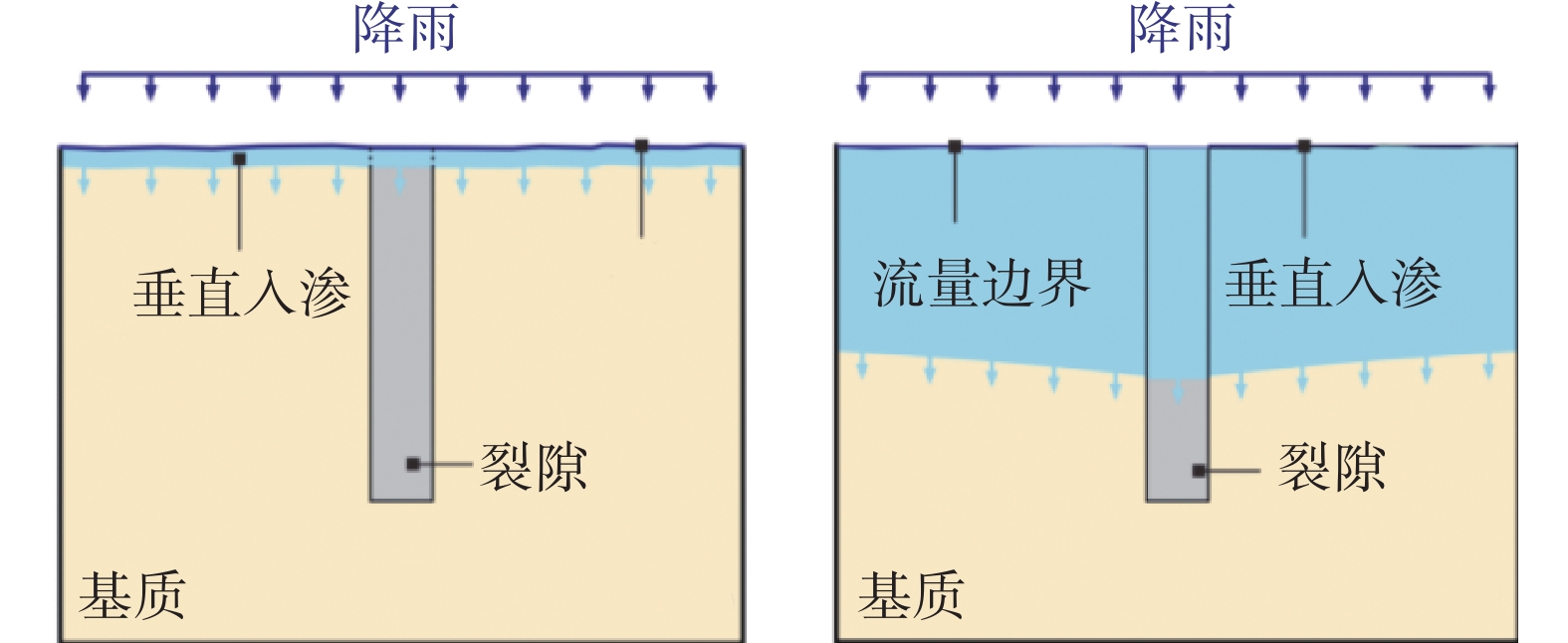
优势流入渗过程示意图如图15、16。短时强降雨作用下,在降雨初期(图15.b),基质与裂隙的入渗能力都较强,此时降雨可全部入渗,基质与裂隙入渗边界均为流量边界,入渗量受降雨强度控制,此时降雨入渗为土体基质控制的垂直入渗,浸润锋总体表现为“水平型”。随着降雨持续(图15.c),基质表面饱和出现积水,降雨强度超过基质入渗能力,基质表面的入渗边界将转化为压力入渗边界,而裂隙的入渗能力较大,裂隙表面的入渗边界仍为流量边界,并且超过基质入渗能力的降水也将由优势的表面入渗。此时大部分为裂隙控制的水平入渗,浸润锋总体表现为“水滴型”。降雨持续一段时间后(图15.d),在基质垂直入渗与裂隙优势流入渗的共同影响下,裂隙影响范围内的土体达到饱和,坡面开始出现径流,裂隙域与基质域均转化为积水入渗边界。此时降雨入渗为土体基质控制的垂直入渗,浸润锋总体表现为“水平型”。长时弱降雨作用下,降雨在基质和裂隙中的入渗作用均较强,降雨可全部入渗,入渗边界为流量边界,入渗量受降雨强度控制。并且降雨在裂隙和土体基质中的入渗速度相差不大,此时降雨入渗为土体基质控制的垂直入渗,浸润锋总体表现为“水平型”,如图16.b-图16.c
以徐洼滑坡为例,研究表明,累积位移较大的监测点附近明显的地表裂隙形成的优势流通道是此类滑坡失稳的关键因素。在短时强降雨条件下,随着裂隙的出现与发育,入渗量逐渐提高,雨水入渗逐渐转变为受优势流控制的优势流入渗[31 − 32]。与本文数值模拟分析结果结果一致。
以粘土为主的膨胀土滑坡其滑体饱和渗透系数较小,在降雨作用下,雨水只有小部分入渗进入坡体,大部分降雨转化为坡面径流排泄[32],降雨入渗的深度有限,理论上不易发生失稳破坏。但本文研究认为,因膨胀土粘土矿物含量高,对水分变化十分敏感,易吸水膨胀失水收缩,因此膨胀土易产生裂隙,裂隙发育形成的各类优势流通道控制膨胀土滑坡发生失稳破坏的关键因素。受膨胀土滑坡土质性质的影响,滑坡表面分布大量裂隙,裂隙为雨水入渗提供了通道,而裂隙末端土体完整性较好,渗透性较差,在强降雨或者持续降雨作用下,容易在此位置形成暂饱和区,构成膨胀土土体内部的潜在滑动面。研究膨胀土中存在的各类优势入渗通道的渗流特征,以及优势流持续作用下滑坡的稳定性演化规律,对深化认识膨胀土滑坡触发和变形破坏机制,优化该类灾害的预警模型,具有较高的理论意义和工程实用价值。
4. 结论
(1)裂隙处与无裂隙处的入渗模式不同,无裂隙处降雨主要为沿土柱表面向下的垂直入渗,饱和方式为由上向下饱和,浸润锋表现为“直线型”下移。裂隙处降雨首先充满裂隙,在裂隙末端形成暂饱和区,随后向两侧水平入渗,饱和方式为由下向上饱和,浸润锋表现为“漏斗型”—“水滴型”—“直线型”。
(2)短时强降雨作用下,随着裂隙深度增加,优势流控制着降雨入渗过程,入渗量和入渗深度与裂隙深度呈正比,入渗深度与裂隙深度基本一致。并且延长了径流产生以及表层土体受冲刷的时间,而随着深层土体达到饱和,不利于斜坡整体稳定。
(3)长时弱降雨作用下,优势流入渗效果减弱,优势流现象产生较慢,降雨沿裂隙入渗速度与在基质孔隙入渗速度基本一致,入渗主要表现为沿土柱表面向下的垂直入渗。入渗量和入渗深度增幅较小,在相同降雨时间内,最终入渗量和入渗深度对裂隙深度的变化不显著。
-
表 1 数值模拟计算参数
Table 1 Summary of simulation model parameters
岩土性质 本构模型 容重/(kN/m3) 粘聚力/kPa 内摩擦角/(°) 弹性模量/MPa 泊松比 填料 摩尔库伦 22.5 50 35 60 0.30 高挡墙 线弹性 24.0 / / 28000 0.20 岩溶充填物 摩尔库伦 18.2 20 13 10 0.32 灰岩 摩尔库伦 26.5 200 40 10000 0.25 灰岩层面 摩尔库伦 / 60 25 / / 节理面 摩尔库伦 / 20 35 / / 挡墙-灰岩接触面 摩尔库伦 / 0 35 / / 挡墙-填料接触面 摩尔库伦 / 0 30 / / 挡墙-岩溶充填物接触面 摩尔库伦 / 0 15 / / 填料-灰岩接触面 摩尔库伦 / 45 35 / / -
[1] 王双,王睿,邱存家,等. 某机场高填方边坡变形机理及支护设计研究[J]. 工程地质学报,2018,26 (增刊1):248 − 253. [WANG Shuang,WANG Rui,QIU Cunjia,et al. Research on deformation mechanism and support design of high fill slope in an airfield[J]. Journal of Engineering Geology,2018,26 (Sup S1):248 − 253. (in Chinese with English abstract)] WANG Shuang, WANG Rui, QIU Cunjia, et al. Research on deformation mechanism and support design of high fill slope in an airfield[J]. Journal of Engineering Geology, 2018, 26 (Sup S1): 248 − 253. (in Chinese with English abstract)
[2] 邱存家,胡勇生,王双,等. 孔内深层强夯桩处理软弱地基试验[J]. 公路交通科技(应用技术版),2018,14(12):70 − 73. [QIU Cunjia,HU Yongsheng,WANG Shuang,et al. Test of treating soft foundation with deep dynamic compaction pile in hole[J]. Journal of Highway and Transportation Research and Development:Application Technical Edition,2018,14(12):70 − 73. (in Chinese)] QIU Cunjia, HU Yongsheng, WANG Shuang, et al. Test of treating soft foundation with deep dynamic compaction pile in hole[J]. Journal of Highway and Transportation Research and Development: Application Technical Edition, 2018, 14(12): 70 − 73. (in Chinese)
[3] 廖鸿,徐超,杨阳. 某机场飞行区土工格栅加筋高边坡优化设计[J]. 水文地质工程地质,2021,48(6):113 − 121. [LIAO Hong,XU Chao,YANG Yang. Optimal design of the high geogrid-reinforced slope at the airfield of an airport[J]. Hydrogeology & Engineering Geology,2021,48(6):113 − 121. (in Chinese with English abstract)] LIAO Hong, XU Chao, YANG Yang. Optimal design of the high geogrid-reinforced slope at the airfield of an airport[J]. Hydrogeology & Engineering Geology, 2021, 48(6): 113 − 121. (in Chinese with English abstract)
[4] 刘许锋. 重力式挡土墙设计与计算在公路工程中的应用[J]. 建筑技术开发,2017,44(12):110 − 111. [LIU Xufeng. Application of gravity retaining wall design and calculation in highway engineering[J]. Building Technology Development,2017,44(12):110 − 111. (in Chinese with English abstract)] DOI: 10.3969/j.issn.1001-523X.2017.12.076 LIU Xufeng. Application of gravity retaining wall design and calculation in highway engineering[J]. Building Technology Development, 2017, 44(12): 110 − 111. (in Chinese with English abstract) DOI: 10.3969/j.issn.1001-523X.2017.12.076
[5] 邵龙潭,刘士乙,李红军. 基于有限元滑面应力法的重力式挡土墙结构抗滑稳定分析[J]. 水利学报,2011,42(5):602 − 608. [SHAO Longtan,LIU Shiyi,LI Hongjun. Analysis of stability against sliding for gravity retaining wall structure based on finite element slip surface stress method[J]. Journal of Hydraulic Engineering,2011,42(5):602 − 608. (in Chinese with English abstract)] SHAO Longtan, LIU Shiyi, LI Hongjun. Analysis of stability against sliding for gravity retaining wall structure based on finite element slip surface stress method[J]. Journal of Hydraulic Engineering, 2011, 42(5): 602 − 608. (in Chinese with English abstract)
[6] VARGA R,ŽLENDER B,JELUŠIČ P. Multiparametric analysis of a gravity retaining wall[J]. Applied Sciences,2021,11(13):6233. DOI: 10.3390/app11136233
[7] 中华人民共和国住房和城乡建设部. 建筑边坡工程技术规范:GB 50330—2013[S]. 北京:中国建筑工业出版社,2014. [Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Technical code for building slope engineering:GB 50330—2013[S]. Beijing:China Architecture & Building Press,2014. (in Chinese)] Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Technical code for building slope engineering: GB 50330—2013[S]. Beijing: China Architecture & Building Press, 2014. (in Chinese)
[8] 张彦伟,宋星光. 贵阳首个超高重力式挡土墙施工技术[J]. 山西建筑,2011,37(35):141 − 143. [ZHANG Yanwei,SONG Xingguang. On construction technique of the first super-high gravity retaining walls in Guiyang[J]. Shanxi Architecture,2011,37(35):141 − 143. (in Chinese with English abstract)] DOI: 10.3969/j.issn.1009-6825.2011.35.084 ZHANG Yanwei, SONG Xingguang. On construction technique of the first super-high gravity retaining walls in Guiyang[J]. Shanxi Architecture, 2011, 37(35): 141 − 143. (in Chinese with English abstract) DOI: 10.3969/j.issn.1009-6825.2011.35.084
[9] 朱向东. 超高挡土墙结构设计[J]. 建筑结构,2010,40(7):109 − 111. [ZHU Xiangdong. Structure design of a super-high retaining wall[J]. Building Structure,2010,40(7):109 − 111. (in Chinese with English abstract)] ZHU Xiangdong. Structure design of a super-high retaining wall[J]. Building Structure, 2010, 40(7): 109 − 111. (in Chinese with English abstract)
[10] 左莎,吴丙贵. 超高挡土墙结构技术分析与经济比较[J]. 水运工程,2002(11):29 − 31. [ZUO Sha,WU Binggui. Technical analysis and economic comparison of ultra-high retaining wall structures[J]. Port & Waterway Engineering,2002(11):29 − 31. (in Chinese with English abstract)] DOI: 10.3969/j.issn.1002-4972.2002.11.009 ZUO Sha, WU Binggui. Technical analysis and economic comparison of ultra-high retaining wall structures[J]. Port & Waterway Engineering, 2002(11): 29 − 31. (in Chinese with English abstract) DOI: 10.3969/j.issn.1002-4972.2002.11.009
[11] 王柳江,赵志杰,刘斯宏,等. 宜兴抽水蓄能电站上库主坝重力挡墙位移影响因素分析[J]. 河海大学学报(自然科学版),2021,49(4):358 − 365. [WANG Liujiang,ZHAO Zhijie,LIU Sihong,et al. Analysis of influence factors on gravity retaining wall displacement of main dam for the upper-reservoir of Yixing Pumped-Storage Hydropower Station[J]. Journal of Hohai University (Natural Sciences),2021,49(4):358 − 365. (in Chinese with English abstract)] WANG Liujiang, ZHAO Zhijie, LIU Sihong, et al. Analysis of influence factors on gravity retaining wall displacement of main dam for the upper-reservoir of Yixing Pumped-Storage Hydropower Station[J]. Journal of Hohai University (Natural Sciences), 2021, 49(4): 358 − 365. (in Chinese with English abstract)
[12] 杨健,刘斯宏,杨建洲. 宜兴抽水蓄能电站高重力挡墙稳定性综合评价[J]. 岩石力学与工程学报,2007,26(增刊2):4439 − 4445. [YANG Jian,LIU Sihong,YANG Jianzhou. Comprehensive evaluation of stability of high gravity retaining wall in Yixing pumped storage power station[J]. Chinese Journal of Rock Mechanics and Engineering,2007,26(Sup 2):4439 − 4445. (in Chinese)] YANG Jian, LIU Sihong, YANG Jianzhou. Comprehensive evaluation of stability of high gravity retaining wall in Yixing pumped storage power station[J]. Chinese Journal of Rock Mechanics and Engineering, 2007, 26(Sup 2): 4439 − 4445. (in Chinese)
[13] 江松笙,康海冬. 宜兴抽水蓄能电站上库主坝高挡墙土压力分析[J]. 岩土力学,2006,27(S2):125 − 131. [JIANG Songsheng,KANG Haidong. Analysis of pressures on high retaining wall earth pressure of main dam of upper reservoir in Yixing pumped storage power station[J]. Rock and Soil Mechanics,2006,27(S2):125 − 131. (in Chinese with English abstract)] JIANG Songsheng, KANG Haidong. Analysis of pressures on high retaining wall earth pressure of main dam of upper reservoir in Yixing pumped storage power station[J]. Rock and Soil Mechanics, 2006, 27(S2): 125 − 131. (in Chinese with English abstract)
[14] 曹贤发,刘之葵,李海玲. 岩溶区建筑场地桩基平均入岩高程预测[J]. 水文地质工程地质,2019,46(4):119 − 125. [CAO Xianfa,LIU Zhikui,LI Hailing. An analysis of the average entering-rock height of grouped piles at building sites in a Karst terrain[J]. Hydrogeology & Engineering Geology,2019,46(4):119 − 125. (in Chinese with English abstract)] CAO Xianfa, LIU Zhikui, LI Hailing. An analysis of the average entering-rock height of grouped piles at building sites in a Karst terrain[J]. Hydrogeology & Engineering Geology, 2019, 46(4): 119 − 125. (in Chinese with English abstract)
[15] 肖鸿斌,金耀岷. 深埋串珠状溶洞的超高层基础设计案例分析[J]. 中国岩溶,2023,42(2):382 − 390. [XIAO Hongbin,JIN Yaomin. Case study on super high-rise foundation design of the deep-buried beaded Karst cave[J]. Carsologica Sinica,2023,42(2):382 − 390. (in Chinese with English abstract)] DOI: 10.11932/karst20230209 XIAO Hongbin, JIN Yaomin. Case study on super high-rise foundation design of the deep-buried beaded Karst cave[J]. Carsologica Sinica, 2023, 42(2): 382 − 390. (in Chinese with English abstract) DOI: 10.11932/karst20230209
[16] 姜巽,曹聪,刘智,等. 歌乐山地区隧道工程诱发的岩溶塌陷发育规律与形成条件[J]. 水文地质工程地质,2023,50(5):181 − 191. [JIANG Xun,CAO Cong,LIU Zhi,et al. Development and formation conditions of Karst collapse induced by tunnel engineering in the Gele Mountain Area[J]. Hydrogeology & Engineering Geology,2023,50(5):181 − 191. (in Chinese with English abstract)] JIANG Xun, CAO Cong, LIU Zhi, et al. Development and formation conditions of Karst collapse induced by tunnel engineering in the Gele Mountain Area[J]. Hydrogeology & Engineering Geology, 2023, 50(5): 181 − 191. (in Chinese with English abstract)
[17] 康晓波,王宇,张华,等. 云南高原岩溶塌陷发育特征及成因机制[J]. 中国地质灾害与防治学报,2022,33(5):50 − 58. [KANG Xiaobo,WANG Yu,ZHANG Hua,et al. Characteristics and formation mechanism of Karst collapse in Yunnan Plateau[J]. The Chinese Journal of Geological Hazard and Control,2022,33(5):50 − 58. (in Chinese with English abstract)] KANG Xiaobo, WANG Yu, ZHANG Hua, et al. Characteristics and formation mechanism of Karst collapse in Yunnan Plateau[J]. The Chinese Journal of Geological Hazard and Control, 2022, 33(5): 50 − 58. (in Chinese with English abstract)
[18] 刘自强,马洪生,牟云娟. 节理裂隙发育岩溶地基数值模拟稳定性分析[J]. 中国岩溶,2022,41(1):100 − 110. [LIU Ziqiang,MA Hongsheng,MOU Yunjuan. Numerical simulation analysis and evaluation of stability of the Karst foundation with developed joints and fissures[J]. Carsologica Sinica,2022,41(1):100 − 110. (in Chinese with English abstract)] DOI: 10.11932/karst20220105 LIU Ziqiang, MA Hongsheng, MOU Yunjuan. Numerical simulation analysis and evaluation of stability of the Karst foundation with developed joints and fissures[J]. Carsologica Sinica, 2022, 41(1): 100 − 110. (in Chinese with English abstract) DOI: 10.11932/karst20220105
[19] 钟祖良,王南云,李滨,等. 采动作用下上硬下软型缓倾岩质高边坡变形机理试验研究[J]. 中国岩溶,2020,39(4):509 − 517. [ZHONG Zuliang,WANG Nanyun,LI Bin,et al. Experimental study on the deformation mechanism of upper-hard and lower-soft gently dipping rock on high slopes under the mining effect[J]. Carsologica Sinica,2020,39(4):509 − 517. (in Chinese with English abstract)] ZHONG Zuliang, WANG Nanyun, LI Bin, et al. Experimental study on the deformation mechanism of upper-hard and lower-soft gently dipping rock on high slopes under the mining effect[J]. Carsologica Sinica, 2020, 39(4): 509 − 517. (in Chinese with English abstract)
[20] 马昊,黄达,肖衡林,等. 江北机场高填方夯后碎块石土剪切力学性质研究[J]. 水文地质工程地质,2019,46(3):88 − 94. [MA Hao,HUANG Da,XIAO Henglin,et al. A study of the shear mechanical properties of high-filled gravel-block soil after dynamic compaction near the Jiangbei Airport[J]. Hydrogeology & Engineering Geology,2019,46(3):88 − 94. (in Chinese with English abstract)] MA Hao, HUANG Da, XIAO Henglin, et al. A study of the shear mechanical properties of high-filled gravel-block soil after dynamic compaction near the Jiangbei Airport[J]. Hydrogeology & Engineering Geology, 2019, 46(3): 88 − 94. (in Chinese with English abstract)
[21] 马翔,赖国泉. 某山区机场高填方滑坡变形特征分析[J]. 中国地质灾害与防治学报,2019,30(4):16 − 23. [MA Xiang,LAI Guoquan. Analysis on deformation monitoring of a high fill landslide in a mountain airport[J]. The Chinese Journal of Geological Hazard and Control,2019,30(4):16 − 23. (in Chinese with English abstract)] MA Xiang, LAI Guoquan. Analysis on deformation monitoring of a high fill landslide in a mountain airport[J]. The Chinese Journal of Geological Hazard and Control, 2019, 30(4): 16 − 23. (in Chinese with English abstract)
[22] 陶小虎,叶明,龚建师,等. 基于LBM-DEM细观数值模拟的水力诱导覆盖型岩溶地面塌陷发育过程分析[J]. 中国地质灾害与防治学报,2024,35(1):124 − 131. [TAO Xiaohu,YE Ming,GONG Jianshi,et al. Analysis of the formation process of the covered Karst ground collapse induced by groundwater changes based on the coupled LBM-DEM numerical simulation at micro scale[J]. The Chinese Journal of Geological Hazard and Control,2024,35(1):124 − 131. (in Chinese with English abstract)] TAO Xiaohu, YE Ming, GONG Jianshi, et al. Analysis of the formation process of the covered Karst ground collapse induced by groundwater changes based on the coupled LBM-DEM numerical simulation at micro scale[J]. The Chinese Journal of Geological Hazard and Control, 2024, 35(1): 124 − 131. (in Chinese with English abstract)
[23] ZHOU Hang,LIU Hanlong,LI Xueyuan,et al. Plasticity solution for the limit vertical pressure of a single rigid pile with a pile cap in soft soil[J]. Computers and Geotechnics,2020,117:103260. DOI: 10.1016/j.compgeo.2019.103260
[24] LI Shiqi,YANG Zhongping,TIAN Xin,et al. Influencing factors of scale effects in large-scale direct shear tests of soil-rock mixtures based on particle breakage[J]. Transportation Geotechnics,2021,31:100677. DOI: 10.1016/j.trgeo.2021.100677
[25] YANG Zhongping,LI Shiqi,JIANG Yuanwen,et al. Shear mechanical properties of the interphase between soil–rock mixtures and benched bedrock slope surfaces[J]. International Journal of Geomechanics,2022,22(5):04022047. DOI: 10.1061/(ASCE)GM.1943-5622.0002342
[26] 中华人民共和国住房和城乡建设部. 工程岩体分级标准:GB/T 50218—2014[S]. 北京:中国计划出版社,2015. [Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Standard for engineering classification of rock mass:GB/T 50218—2014[S]. Beijing:China Planning Press,2015. (in Chinese)] Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Standard for engineering classification of rock mass: GB/T 50218—2014[S]. Beijing: China Planning Press, 2015. (in Chinese)
[27] 汪小刚,林兴超. 基于刚性块体离散的边坡稳定极限分析法[J]. 岩土工程学报,2022,44(9):1587 − 1597. [WANG Xiaogang,LIN Xingchao. Limit analysis method for slope stability based on discretization of rigid blocks[J]. Chinese Journal of Geotechnical Engineering,2022,44(9):1587 − 1597. (in Chinese with English abstract)] DOI: 10.11779/CJGE202209003 WANG Xiaogang, LIN Xingchao. Limit analysis method for slope stability based on discretization of rigid blocks[J]. Chinese Journal of Geotechnical Engineering, 2022, 44(9): 1587 − 1597. (in Chinese with English abstract) DOI: 10.11779/CJGE202209003
[28] 李志浩,肖世国. 地震条件下悬臂式挡墙主动土压力的极限分析方法[J]. 中国地质灾害与防治学报,2020,31(5):79 − 87. [LI Zhihao,XIAO Shiguo. Limit analysis method of active earth pressure on cantilever retaining wall subjected to earthquake[J]. The Chinese Journal of Geological Hazard and Control,2020,31(5):79 − 87. (in Chinese with English abstract)] LI Zhihao, XIAO Shiguo. Limit analysis method of active earth pressure on cantilever retaining wall subjected to earthquake[J]. The Chinese Journal of Geological Hazard and Control, 2020, 31(5): 79 − 87. (in Chinese with English abstract)
[29] 中国民用航空局. 民用机场岩土工程设计规范:MH/T 5027—2013[S]. 北京:中国民航出版社,2013. [Civil Aviation Administration of China Design Code for Civil Airport Geotechnical Engineering:MH/T 5027-2013[S]. Beijing:Civil Aviation Press of China,2013. Self service filling. (in Chinese )] Civil Aviation Administration of China Design Code for Civil Airport Geotechnical Engineering: MH/T 5027-2013[S]. Beijing: Civil Aviation Press of China, 2013. Self service filling. (in Chinese )




 下载:
下载:
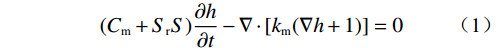
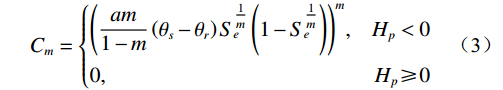

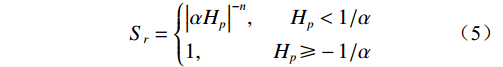
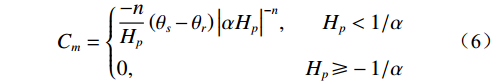
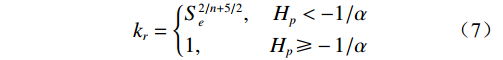
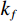
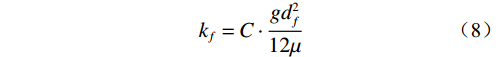

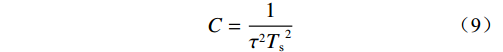
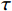
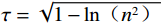


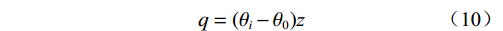

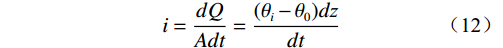
















 邮件订阅
邮件订阅 RSS
RSS